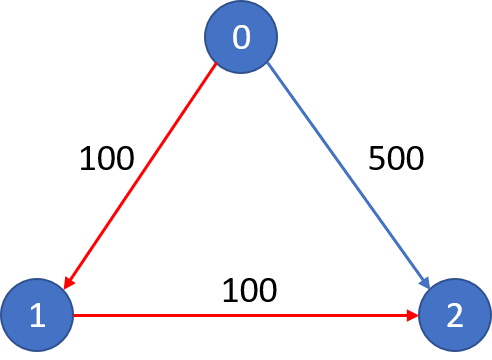
There are n cities connected by m flights. Each flight starts from city u and arrives at v with a price w.
Now given all the cities and flights, together with starting city src and the destination dst, your task is to find the cheapest price from src to dst with up to k stops. If there is no such route, output -1.
Example 1: Input: n = 3, edges = [[0,1,100],[1,2,100],[0,2,500]] src = 0, dst = 2, k = 1 Output: 200 Explanation: The graph looks like this:The cheapest price from city
0to city2with at most 1 stop costs 200, as marked red in the picture.
Example 2: Input: n = 3, edges = [[0,1,100],[1,2,100],[0,2,500]] src = 0, dst = 2, k = 0 Output: 500 Explanation: The graph looks like this:The cheapest price from city
0to city2with at most 0 stop costs 500, as marked blue in the picture.
Constraints:
- The number of nodes
nwill be in range[1, 100], with nodes labeled from0ton- 1. - The size of
flightswill be in range[0, n * (n - 1) / 2]. - The format of each flight will be
(src,dst, price). - The price of each flight will be in the range
[1, 10000]. kis in the range of[0, n - 1].- There will not be any duplicated flights or self cycles.
Related Topics:
Dynamic Programming, Heap, Breadth-first Search
Similar Questions:
With k stops, the longest path has k + 1 edges. So, using Bellman-ford algorithm, we should relax k + 1 times.
The relaxation happens from layer to layer. This is to prevent using distance in layer i when relaxing from layer i-1 to layer i.
// OJ: https://leetcode.com/problems/cheapest-flights-within-k-stops/
// Author: github.com/lzl124631x
// Time: O(K * (N+ E))
// Space: O(N)
class Solution {
public:
int findCheapestPrice(int n, vector<vector<int>>& A, int src, int dst, int K) {
vector<int> dist(n, 1e8);
dist[src] = 0;
for (int i = 0; i <= K; ++i) {
auto tmp = dist;
for (auto &e : A) {
int u = e[0], v = e[1], w = e[2];
dist[v] = min(dist[v], tmp[u] + w);
}
}
return dist[dst] == 1e8 ? -1 : dist[dst];
}
};// OJ: https://leetcode.com/problems/cheapest-flights-within-k-stops/
// Author: github.com/lzl124631x
// Time: O(KElogE)
// Space: O(KN + E)
class Solution {
typedef array<int, 3> item; // distance, cityId, # stops left
public:
int findCheapestPrice(int n, vector<vector<int>>& E, int src, int dst, int k) {
vector<vector<pair<int, int>>> G(n);
for (auto &e : E) G[e[0]].emplace_back(e[1], e[2]);
priority_queue<item, vector<item>, greater<>> pq;
++k;
pq.push({0, src, k});
vector<vector<int>> dist(k + 1, vector<int>(n, INT_MAX));
for (int i = 0; i <= k; ++i) dist[i][src] = 0;
while (pq.size()) {
auto [cost, u, stop] = pq.top();
pq.pop();
if (cost > dist[stop][u]) continue;
if (u == dst) return cost;
if (stop == 0) continue;
for (auto &[v, w] : G[u]) {
if (dist[stop - 1][v] > cost + w) {
dist[stop - 1][v] = cost + w;
pq.push({dist[stop - 1][v], v, stop - 1});
}
}
}
return -1;
}
};A plain BFS also works, but it doesn't prioritize low cost paths first so it might be less efficient.
// OJ: https://leetcode.com/problems/cheapest-flights-within-k-stops/
// Author: github.com/lzl124631x
// Time: O(KE)
// Space: O(N + E)
class Solution {
public:
int findCheapestPrice(int n, vector<vector<int>>& E, int src, int dst, int k) {
vector<vector<pair<int, int>>> G(n);
for (auto &e : E) G[e[0]].emplace_back(e[1], e[2]);
queue<pair<int, int>> q; // cityId, cost
q.emplace(src, 0);
vector<int> dist(n, INT_MAX);
dist[src] = 0;
int step = 0;
while (step <= k) {
int cnt = q.size();
while (cnt--) {
auto [u, cost] = q.front();
q.pop();
for (auto &[v, w] : G[u]) {
if (dist[v] > cost + w) { // Note that we shouldn't use `dist[u]` here. Because `dist[u]` might get updated using two jumps but the `cost` here corresponds to one jump.
dist[v] = cost + w;
q.emplace(v, dist[v]);
}
}
}
++step;
}
return dist[dst] == INT_MAX ? -1 : dist[dst];
}
};